KINEMATICS EXAMPLE 7

The motion of a car on a straight road is given by the relationship:

 

           

 

The road is oriented North-South with North as the positive direction, and locations are measured relative to where the road dead-ends with an East-West road at its North end.  Describe and represent this car’s motion in as many ways as you can.

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

Since the equation, we are given “maps” onto the general equation for an object undergoing a constant acceleration motion, i.e.,

 

           

we see that  and   This tells us that the car started at a point .23 km South of the dead-end, moving North at 2 m/s, and that it is accelerating to the North.  Since the velocity and the acceleration are in the same direction, the car is speeding up.  So, the motion map looks like:

 

 

 

 

 

 

 

 

 

 

 

To draw the position map we need to actually find the car’s positions at specific instants.  Doing the calculations gives us the chart below:

 

 

 

 

 

 

 

 

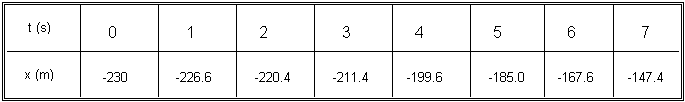
Now we can draw the position map for the car.  We will draw the positions from the car’s initial location using a scale of 1 cm on the paper equals 10 m in the actual situation.  The position map looks like:

 

 

 

 

 

 

 

 

 

 

 

 

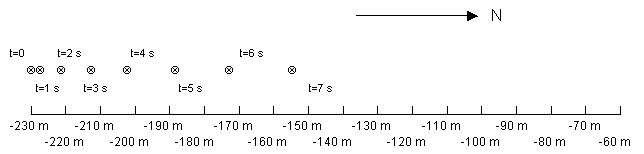
Now that we have the position map, we can readily draw the position versus time graph.

We can also draw the velocity versus time graph for this car.  It will be a straight line starting at the point (0,2 m/s) and having a slope of 2.8 m/s/s.  This is shown on Graph B.

 

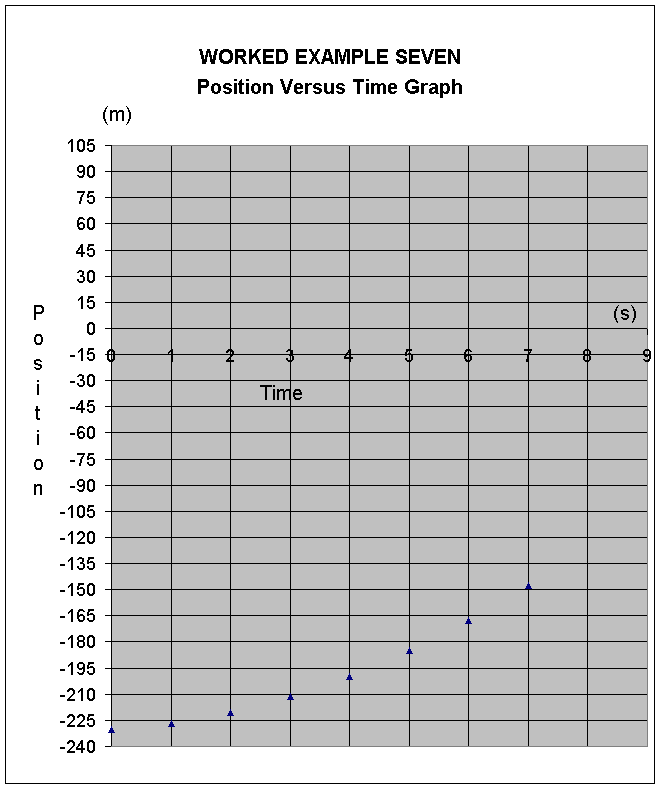
Graph A

 

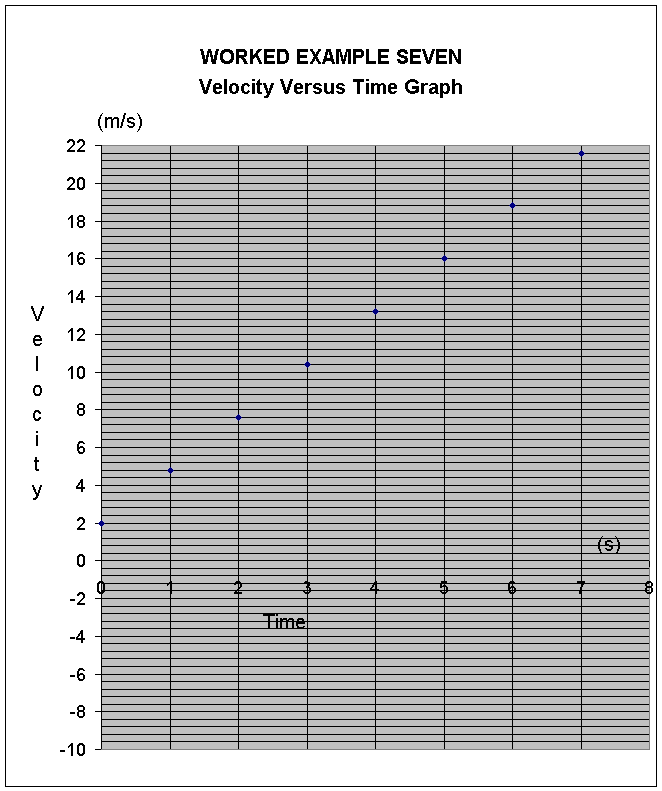
Graph B