KINEMATICS EXAMPLE 6

 

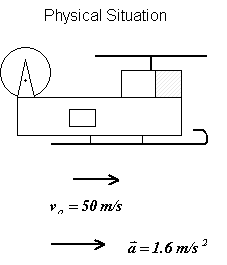
A helicopter, traveling at 180 km/hr accelerates at 1.6 m/s² for 15 sec.  What is its speed at the end of the 15 sec, and how far did it travel during that time?  Determine the distance at least two different ways.

 

Given:             initial speed, v_o = 180 km/hr = 50 m/s;

                        acceleration (assumed constant), a = 1.6 m/s²;

                        time interval, Dt = 15 sec.

 

Unknown:       Speed at the end of the 15 sec. interval, i.e., final speed, v_f = ? m/s.

                        Distance traveled during the 15 sec, d = ? m.

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

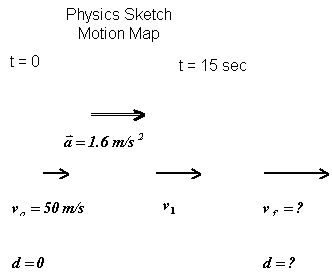
Physical (Mathematical) Principles and/or Ideas:  relation for speed and acceleration in uniformly accelerated motion, relation for distance traveled during uniformly accelerated motion.

 

Solution:         This problem is rather straightforward.  We know the initial speed, the acceleration, and how long the helicopter accelerates, and we are looking for the final speed at the end of the period of acceleration.  Since acceleration is the rate of change of velocity, which if the object is traveling in a straight line, AND ONLY THEN, reduces to the rate of change of speed, we can quickly write an equation:

                                                           

 

There are four quantities in this equation; we know three of the four, and the fourth is our unknown.  Solving for the final speed gives:

 

                                                           

 

Putting in the given values and doing the math, we get:

 

           

 

There several ways to find the distance the helicopter travels during the 15 sec.  One way would be to calculate the helicopter’s average speed, which we could do now that we know both the initial and final speeds, and multiply the average speed by the time.  Doing this we get:

 

                       

                       

 

Another way to get this distance is to use the relation for distance when an object is undergoing uniformly accelerated motion.  That is:

 

           

 

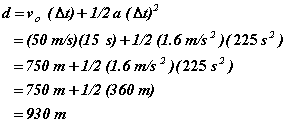
Notice that we get the same answer either way, which we must if we do both properly.  Notice also that the two terms on the right of the relation above  and  

-- both are distances!  Why is this necessary?

 

What relation, if any, does the problem we just worked have to the problem below?

 

A speedboat covers a distance of 685 m in 22 seconds.  If the boat started out going 24 m/s, what was its acceleration and how fast was it going at the end of 22 seconds?

 

How are the two problems similar?  How do they differ?

How does the behavior of the boat compare to that of the helicopter?

Is there any fundamental physical difference in the motion of the boat compared to the motion of the helicopter?