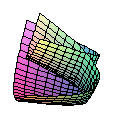|
|
Click on the small picture to see the larger picture.
Click on the  square to load an animated gif.
|
|
An article of K. Boyadzhiev in the September 2007 issue of The
College Mathematics Journal proposes a notion of an "Equiangular
surface." The picture links to a page showing two pictures of an
example from that paper.
|
|
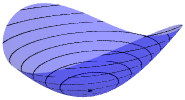
The picture links to a page about surfaces which are graphs of
functions with critical points. This is also related to
my Memoir, Unfolding
CR Singularities.
|
|
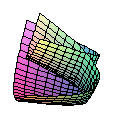
The picture links to a page about affine surfaces parametrized by
quadratic polynomials.
|
302KB
gif
|
The picture illustrates some of the domains that appear in a joint paper on
A Taylor series condition for harmonic extension.
|
1,145KB
gif
POV
scene file
POV
ini file
|
The picture links to a page about the hippopede of Proclus
and some related special plane curves.
|
|
The picture links to a page about linear systems of conics.
|
|
The picture links to a page about linear systems of
ellipsoids.
I've given a couple related math club talks about ellipsoid packing: 2008 Talk, 2004 Talk
|
231KB
gif
POV
scene file
POV
ini file
|
A deflating ellipsoid. The animation demonstrates the
ellipsoids
from Examples 4.6 and 4.7 of my paper on
CR singular immersions of complex projective spaces. The two
points on the ellipsoid that don't move during the animation,
(0,0,1) and (0,0,-1), have tangent planes parallel to the
xy-plane (the wall on the left). The "Bishop invariant" at
these points starts with value 0, when the surface is a sphere, and
increases, with upper bound 1/2, until the surface flattens into an
elliptical disc in the yz-plane.
This animation is also an example of a deformation of an elliptic
point, as described in my paper on Unfolding CR
singularities.
Yet another phenomenon demonstrated by this sequence is how
a Steiner
surface can collapse into two dimensions. At t = 0 (the
first frame of the animation, shown in the still picture), these
parametric equations (below) define a sphere. For 0 < t < 1,
the image is an ellipsoid. As t increases to 1, the
parametric component
2(1-t)u0u1 goes to zero,
so at t = 1, the image is contained in the yz-plane.
The implicit equation changes from an ellipsoid for t < 1, to
the entire yz-plane at t = 1. The animation continues
by decreasing the parameter t back to 0, re-inflating the
ellipsoid.
- real quadratic homogeneous parametrization:
[u02+u12+u22
: 2(1-t)u0u1 :
2(-1-t)u0u2 :
-u02+u12+u22)]
- affine implicit equation:
(1+t)2x2+(1-t)2y2+(1-t2)2z2-(1-t2)2
= 0
|
|
This is the Whitney umbrella, a ruled surface. You can see
the "double line," where the surface intersects itself, and the "pinch
point," where the double line terminates in a singularity. The lines
on the surface actually extend indefinitely, so this picture only
shows the part of the object inside a bounding box.
Using real parameters u, v, the surface can be
described by the quadratic parametric equations
(x,y,z)=(u,v2,uv),
so...
Whitney's umbrella can also be described as a cubic variety, since
the points (x,y,z) on the surface satisfy the
implicit equation x2y - z2
= 0. However, all points of the form (0,y,0) satisfy this
equation, so the solution set of the cubic includes the entire double
line (the
y-axis is the handle of the umbrella), instead of just the
closed ray of self-intersection (where u=0
and y=v2 is nonnegative). In the POV code, I
used the cubic implicit equation, but clipped off the negative
y-axis by the bounding box.
|
|
This picture shows a Roman surface, together with two
objects with a similar shape. It is intended to aid in the
visualization of this type
of Steiner
surface, with its six pinch points, three double lines, and one
triple point. The Euclidean volume and surface area of the three
objects are also compared, but other Roman surfaces related to this
one by a linear transformation will of course have different values
for volume and area. The polyhedral shape is called a Reinhardt
heptahedron or a tetrahemihexahedron.
|
916KB
gif
|
More Roman surfaces.
|
 |
The picture links to the "Boy Surface" section on my Steiner
surface page. There is a larger picture of the Boy Surface and
also an animation showing a deformation of Steiner's Roman Surface
into the Boy surface.
|
|
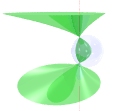
The picture links to a page about the surfaces of Gerhard
Miehlich.
|
|
This picture shows a hyperboloid and a torus. The
interesting parts are the checker pattern (not all squares!) on the
torus, and the reflection of the doughnut hole in the hyperboloid.
|
|
The "real algebraic variety" associated to a polynomial
p(x,y,z) is the set of all points
(x,y,z) that are solutions of the equation
p(x,y,z) = 0. A "real analytic" function
is defined by an infinite sum of terms, and it can similarly describe
a "real analytic variety." Sometimes using finitely many terms in the
series for an analytic function, to get a polynomial approximation, is
called "chopping off the tail."
The picture links to a demonstration of how the solution sets of
some equations can look
like my cat.
|
302KB
gif
1,145KB
gif
231KB
gif
916KB
gif