John's MA 15400
Assignments for Each Day
Spring 2020

 

Class meeting Topic Assignment


Tuesday, Jan. 14

 

 Section 7.1
Periodic Functions		 Welcome to our class.

Handouts: Ferris Wheel  (See pictures of the London Eye: 1  2 3)
                Syllabus
                Internet Resources (with a link to the General Course Information)

Today's objectives:
 1. Identify if a graph represents a periodic function.
 2. Determine period, amplitude and midline.
 3. Use a graph to find and interpret y if given t or vice versa.

Due: Thursday, January 16
Read: General Course Information, the Syllabus, and, if you have the text, Section 7.1 and 7.2
Do:    If necessary, purchase a TI-84 Plus CE or equivalent and purchase eHW access.
           Note: access is good for one year.
           Practice on eHW Flash Cards: 7.1 Periodic Functions.
           Optional: Section 7.1 -- your choice of 1-17, 23-34 as needed.
           Review eHW Math Background Needed for MA 15400 and eHW Flash Cards for Prerequisite Skills 
           See also Practice Questions to Check Prerequisite Skills Needed for MA 15400 and these Worked out Solutions.
           You should also be proficient in these skills needed for MA 15300.

Prepare for QUIZ 1 next Tuesday, Jan. 21 over prerequisite skills.

Due Friday, January 17, 11:59 pm: E-HW: John's Syllabus- Score 90% or Higher by the Deadline!
Note: You need to get a score of 90% or higher for this eHW by the deadline to open up any future eHW assignments.
You have unlimited attempts.

Due Monday, Jan. 20, 11:59 pm:  E-HW0: General Course Information and Using eHW
Due Monday, Jan. 20, 11:59 pm:  E-HW: Math Background Needed for MA 15400
Due Thursday, Jan. 23, 11:59 pm:  E-HW 01 Sections 7.1 - 7.2
Thursday, Jan. 16 Section 7.2
The Sine Function
(and its Sidekick, Cosine)
Today's objectives:
  1. Sketch the position of a point on a circle of radius r corresponding to a given angle (or value of time or number of revolutions) and give its coordinates
  2. Find angles between 0° and 360° which have the same sine or cosine of a given angle.
  3. Determine in which quadrant an angle lies if given certain conditions.


Do (for practice): 
Flash Card 7.2 Report the coordinates of a point on a circle of radius r given the angle.
Flash Card 7.2 Use the definitions of sine and cosine
Flash Card 7.2 Find angles which have the same sine (or cosine) as a given angle
If you have a text, read Section 7.3 and do Section 7.2 -- 1, 3, 5-31
Click on the photo to enlarge (and download for printing).

Prepare for QUIZ 1 next Tuesday, Jan. 21 over prerequisite skills.
(For practice do eHW Math Background Needed for MA 15400 and review eHW Flash Cards for Prerequisite Skills 
See also Practice Questions to Check Prerequisite Skills Needed for MA 15400 and the Worked out Solutions.)

Due Friday, January 17, 11:59 pm: E-HW: John's Syllabus- Score 90% or Higher by the Deadline!
Note: You need to get a score of 90% or higher for this eHW by the deadline to open up any future eHW assignments.
You have unlimited attempts.

Due Monday, Jan. 20, 11:59 pm:  E-HW0: General Course Information and Using eHW
Due Monday, Jan. 20, 11:59 pm:  E-HW: Math Background Needed for MA 15400
Due Thursday, Jan. 23, 11:59 pm:  E-HW 01 Sections 7.1 - 7.2 
Class meeting Topic Assignment
Tuesday, Jan. 21 Section 7.3
Radians
Today's objectives:
  1. Convert an angle from degrees to radians and vice versa.
  2. Interpret the radian measure of the central angle of a circle of radius r as the number of radius lengths, r, that you need to wrap around the rim of the circle on the arc spanned by the angle.
  3. Understand the relationship between arc length, radius and an angle measure in radians.
    If given two of the arc length s, radius r, or an angle θ, find the third.
The radian measure of an angle θ is the number of radius lengths that a bug would walk on the rim of a circle spanned by the angle.
For a circle of radius r, if s is the arc length the bug walks on the rim, then we have
s = (the radius r) x (# of radius lengths) or
s =  r x θ

Here is a short video of the bug taking a tour around the rim of a circle,
counting radius lengths as he goes. It is a silent movie since bugs can't talk.

Suppose a central angle θ  in a circle of radius r spans an arc of length s. Measure how many radius lengths that the length of the arc is.  This number of radius lengths is the radian measure of the angle θ: we have rθ = s.

Do (for practice): 
Flash Card 7.3 Degrees <=> Radians
Flash Card 7.3 Find points on a circle using radian measure of quadrantals
Flash Card 7.3 Radian Measure as Number of Radius Lengths Around a Circle
Flash Card 7.3 Arc Length of a Sector
If you have a text, read Chapter 7 Skills Refresher, see Section 7.3 -- 1-56 as desired (for practice).

Due Thursday, Jan. 23, 11:59 pm:  E-HW 01 Sections 7.1 - 7.2 
Due Tuesday, Jan. 28, 11:59 pm:  E-HW 02 Section 7.3
 Due Thursday, Jan. 30 - Writing Assignment 1: Bug on a Square Track

For next class, Thursday, Jan. 23, please come prepared with the first two pages of WR1 completed.

Thursday, Jan. 23 Today:  Special Angles Handout: Unit circle with exact values
We worked some problems from Writing Assignment 2 on finding angles in radians (exactly).

Today's objectives:
1. Find exact values of sine and cosine for multiples of 30°, 45°, and 60° or their radian equivalents π/6, π /4, or π /3.
2. Use proportional reasoning to find sides of special triangles.
3. Solve simple trig equations over a requested interval; for example on the interval [0, 2π) find θ if sin θ = 1/2, providing exact values of angles measured in radians when they are multiples of π/6, π /4, or π /3. Be aware of when more than one solution exists! Be able to sketch the angle or angles.

Due: Tuesday, Jan. 28
Do (for practice): 
Flash Card Chapter 7 Skills Refresher Properties of Special Triangles
Flash Card Chapter 7 Skills Refresher Exact Values of Sine and Cosine

If you have a text, see Chapter 7 Skills Refresher  (page 324) -- 1 -31  as desired (for practice).

Due tonight, Thursday, Jan. 23, 11:59 pm:  E-HW 01 Sections 7.1 - 7.2 
Due Tuesday, Jan. 28, 11:59 pm:  E-HW 02 Section 7.3
Due Thursday, Jan. 30, 11:59 pm: E-HW 03 Chapter 7 Skills Refresher
Due Thursday, Jan. 30 - Writing Assignment 1: Bug on a Square Track
Due Tuesday, Feb. 4 - Writing Assignment 2: What's My Angle?

For Tuesday, Jan. 28: QUIZ 2 over 7.1 and 7.2
Class meeting Topic Assignment
Tuesday, Jan. 28 Section 7.4 Graphs of Sine and Cosine Today's objectives:
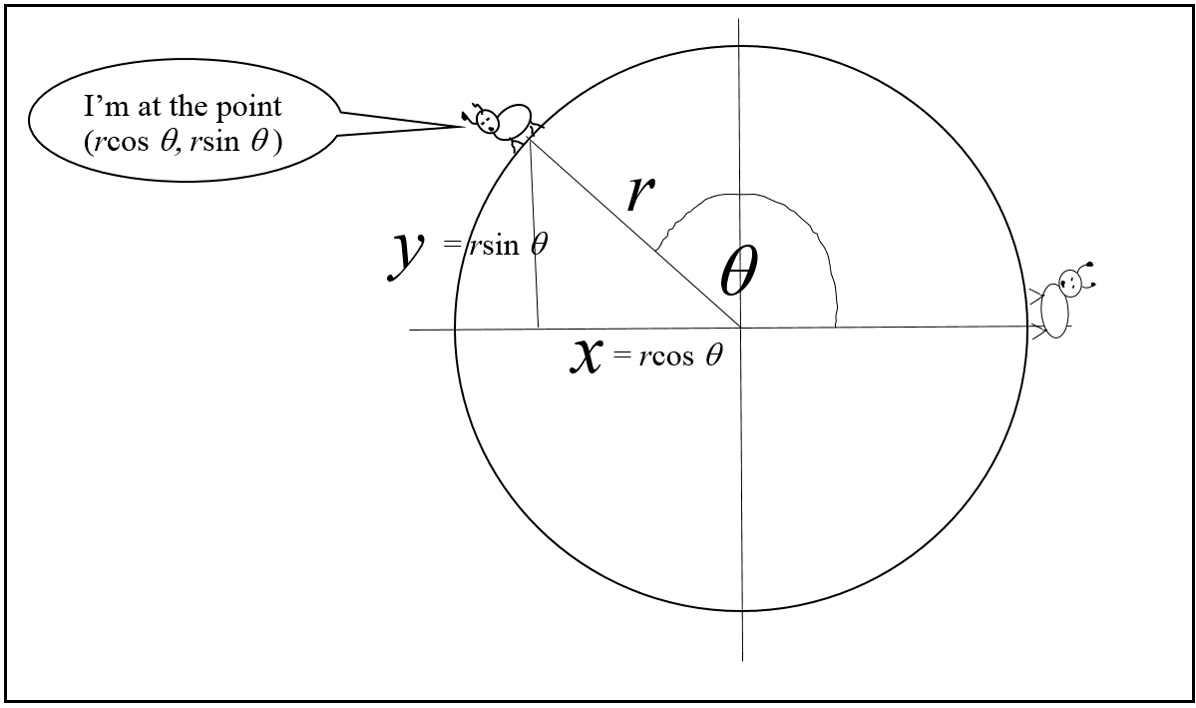
1. Know the main characteristics (period, amplitude, midline, domain, range, odd/even symmetry, when it is positive, negative, increasing, decreasing, if it starts at or above the midline) of the graph of y = sin θ, and y = cos θ. Relate this to the unit circle as the x-coordinate (cosine) or the y-coordinate (sine) of the point on the circle.
2. For y = Asin(x) + k or y = Acos(x) + k , identify the period, amplitude, and midline.

We discussed the domain, range, period, and amplitude of y = sin(x) and y = cos(x) and how these can be determined from the unit circle.
We looked at an outside change to the function, which results in the original function being transformed vertically (change to the output).

  1. y = Asin(x) and y = Acos(x) have amplitude |A|. 
    For A > 0, 
    the graph of y = Asin(x) vertically stretches or compresses the graph of  y = sin(x) by A units.
    the graph of y = –Asin(x) is a vertical reflection of the graph of y = Asin(x).
    Similarly for y = cos(x).  
     

  2. y = sin(x) + k and y = cos(x) + k have midline k.
    For k > 0, 
    the graph of y = sin(x) + k vertically shifts the graph of y = sin(x) up k units.
    the graph of y = sin(x) – k vertically shifts the graph of y = sin(x) down k units.
    Similarly for y = cos(x).  

The first multiplies the output by a quantity; the second adds/subtracts a quantity to the output.
For the function y = Asinx + k or y = Acosx + k
we explored the effects of  A and k to find that this graph has an amplitude of  |A|, and midline which is y = k.

Due: Thursday, Jan. 30
Optional: Section 7.4 -- 1-24, 26-30, 34-38 and Chapter 7 Review 14a, 14d, 19-24, 51, 52, 69-71 and read Section 7.5
Practice on eHW Flash Cards:  7.4 Period, Amplitude, Midline

Due tonight, Tuesday, Jan. 28, 11:59 pm:  E-HW 02 Section 7.3
Due Thursday, Jan. 30, 11:59 pm: E-HW 03 Chapter 7 Skills Refresher
Due Thursday, Jan. 30 - Writing Assignment 1: Bug on a Square Track
Due Tuesday, Feb. 4 - Writing Assignment 2: What's My Angle?
Thursday, Jan. 30 Section 7.5
The graph of
y = sin(Bx)
(Inside multiplicative change)

For the function y = AsinBx + k or y = AcosBx + k
we explored the effects of  B on the period to find that, for positive values of B, this graph has a period of  2π/B.

Today's objectives:
1. Report the period of the graph of y = AsinBx + k.
2. If you have found the period, p, of  y = sinBx, check that your value is correct by substituting into the formula and verifying that B·p = 2π.
3. Given a graph, report the midline, amplitude and period and use them to find the formula y = AsinBx + k or y = AcosBx + k.

Do (for practice):
Flash Card 7.4 Period, Amplitude, Midline
 Flash Card 7.5 Find a Formula of a Sine or Cosine Function (No Phase Shift Needed)
Flash Card 7.5 Find a Formula of a Sine or Cosine Function (Ferris Wheel) - then find y if given x
 Flash Card 7.5 Find a Formula of a Sine or Cosine Function (Ferris Wheel) - then find x if given y
 If you have a text, see Section 7.5 -- 1-12, 21-26  and read Section 7.5 and, if needed, Sections 2.4, 6.1, 6.2
Due tonight, Thursday, Jan. 30, 11:59 pm: E-HW 03 Chapter 7 Skills Refresher

Tuesday, Feb. 4:  QUIZ 3 over Section 7.3 and Chapter 7 Skills Refresher
Due Tuesday, Feb. 4 - Writing Assignment 2: What's My Angle?
Due Tuesday, Feb. 11 - Writing Assignment 3: Trig Graphs
Class meeting Topic Assignment
Tuesday, Feb. 4 Section 7.5
For positive values of
A, B, h, k, and φ
we explored the graph of
y = Asin(B(x − h)) + k or
y = Asin(Bx − φ) + k
where h is the
horizontal shift to the right and
where φ = Bh is the
phase shift to the right		 Today:  QUIZ 3 over 7.3 and Chapter 7 Skills Refresher
Continued Section 7.5. If given a graph, find the midline, period, amplitude, and the horizontal and phase shifts. When specifying horizontal and phase shift, include whether it is regular sine or cosine (or upside down versions of these), whether it is shifted left or right, and by how much.

Today's objectives:
1. Given a graph and a model choice (regular or upside down sine or cosine) report the phase shift.
2. Given a graph with a nonzero phase shift φ or horizontal shift h, find a formula y = Asin(B(x−h))+ k or y = Asin(Bx−φ) + k.
3. If you have found the phase shift, φ, of the graph of y =sin(Bx−φ), check that your value is correct by multiplying out y =sin(B(x−h))
and verifying that B·h = φ, where h is the horizontal shift.

Do (for practice)::
Flash Card 7.5 Find Phase Shift
 Flash Card 7.5 Find a Formula of a Sine or Cosine Function (Phase Shift Needed)        
Optional:  Section 7.5 -- 27-31, 37, 38, 44-50 and read Section 7.6 and, if needed, Sections 2.4, 6.1, 6.2

Due Tuesday, Feb. 11 - Writing Assignment 3: Trig Graphs
Due Tuesday, Feb. 11, 11:59 pm: E-HW 04 Section 7.4-7.5
Thursday, Feb. 6 Section 7.6 The Tangent Function Today's objectives:
1. Know the main characteristics (period, domain, range, symmetry, value at π/4, when it is positive, negative, zero, undefined. increasing)
of the graph of y = tan θ.
2. Relate the graph of y = tan θ to the unit circle as the slope y/x of the line through the origin and the point on the circle.
3. Given the graph of y = AtanBx and its intercepts and vertical asymptotes, find A and B.
4. Given the formula of y = AtanBx, report its intercepts and vertical asymptotes (exact). Solve Atan Bx = A.

Do (for practice):
Flash Card 7.6 Graph of y = AtanBx
If you have a text, see Section 7.6  -- 1-36

Due Tuesday, Feb. 11 - Writing Assignment 3: Trig Graphs
Due Tuesday, Feb. 11, 11:59 pm: E-HW 04 Section 7.4-7.5
Due Thursday, Feb. 20, 11:59 pm:  E-HW 05 Section 7.6 -7.8

 Tuesday, Feb. 11: QUIZ 4 over 7.4 and 7.5
Test 1 next week. See review sheet. This is in the folder Reviews for Exams on Blackboard.
Class meeting Topic Assignment
Tuesday, Feb. 11 The Reciprocal Functions Today's objectives:
1. Find exact values of sin θ, cos θ, tan θ, csc θ, sec θ, cot θ if given the angle θ as a multiple of 30°, 45°, and 60° (or π/6, π/4, or π/3).
2. Find exact values of sin θ, cos θ, tan θ, csc θ, sec θ, cot θ if given the value of one of these trig functions.
 

Do (for practice):
Flash Card 7.8 Solve trig equations for the exact value of the angle WITHOUT a calculator
Flash Card 7.8 Solve trig equations for the approximate value of the angle WITH a calculator
Flash Card 7.8 Given the slope, find the angle of inclination
 

Optional:  Section 7.7  -- 1-20

Due Tuesday, Feb. 20, 11:59 pm:  E-HW 05 Section 7.6 -7.8
 Due Thursday, Feb. 27, 11:59 pm:  E-HW 06 Section 8.1

The test is outside of class in the Math Test Center (KT G18).
Covers: Chapters 7.1 - 7.5, Chapter 7 Skills Refresher, your Writing Assignments WR1, WR2, and WR3 and your past quizzes and eHW assignments.
Walk in with any kind of photo ID and your graphing calculator to take the test.
Math Test Center (KT G18) is open:
     Wed., Feb. 12,  after class - 7:00 PM (it closes at 8:00 pm)
     Thurs., Feb. 13,, 9:00 AM - 7:00 PM (it closes at 8:00 pm)
     Friday, Feb. 14,, 9:00 AM - 3:30 PM (it closes at 4:30 PM)

See the Review Sheet. This is in the folder Reviews for Exams on Blackboard.
Wed., Feb. 12 Walk-in testing available. You can take Test 1 in the Math Test Center (KT G18) Wed., Feb. 12 from  9:00 AM - 7:00 PM.
(It closes at 8:00 pm)
Thursday, Feb. 13 No class meeting
Walk-in testing available.		 No class meeting, (See Review sheet on BB in the folder Reviews for Exams.)
You can take Test 1 in the Math Test Center (KT G18) Thurs., Feb. 13,  9:00 AM-7:00 PM
(It closes at 8:00 pm.)
Friday, Feb. 14 Walk-in testing available. You can take Test 1 in the Math Test Center (KT G18) Fri., Feb. 14,  9:00 AM - 3:30 PM
(It closes at 4:30 pm.)
This is the last day to take the test.
Class meeting Topic Assignment
Tuesday, Feb. 18 Inverse Functions and  Right Triangle Trigonometry with SohCahToa and Pythagoras  Today's objectives:
1. Solve simple trig equations over a requested interval; for example [0, 2π), [0,360°), (−∞,∞), or other intervals, providing
   a. exact values of angles measured in radians (when given special angles as in 1a above)
   b.decimal approximations using the inverse trig functions
2. Distinguish the meaning of the notation sin−1x, arcsin x, sin2x, sin x2, csc x, etc.
3. Interpret tan θ as the slope of the angle of inclination.
4. If given any two of the side lengths of a right triangle, find the remaining parts. 
5. If given a side length and an angle of a right triangle, find the remaining parts. 

Do (for practice):
Flash Card 7.8 Solve trig equations for the exact value of the angle WITHOUT a calculator
Flash Card 7.8 Solve trig equations for the approximate value of the angle WITH a calculator
 Flash Card 8.1 Given the slope, find the angle of inclination
Flash Card 8.1 Use right triangle definitions if given two sides
Flash Card 8.1 Use right triangle definitions if given the angle and a side
Flash Card 8.1 Use right triangle definitions - applications
Optional: If you have a text, see Section 7.8 - - 1-33 and Section 8.1  -- 1-47 as desired

Due Tuesday, Feb. 25, 11:59 pm:  E-HW 05 Section 7.6 -7.8
 Due Tuesday, Feb. 25, 11:59 pm:  E-HW 06 Section 8.1

 Writing Assignment 4: The Gateway Arch due Tuesday, Feb. 25
Thursday, Feb. 20 Solving Triangles Today's objectives:
1. Solve for sides and angles of a triangle using the Law of Sines.
2. Determine when you can use SohCahToa and Pythagoras and when you can use the Law of Sines. 
3. Solve for sides and angles of a triangle using the Law of Cosines.
4. Determine when you can use the Law of Cosines and when you can use the Law of Sines. 

Do (for practice):
Flash Card 8.2 Use Law of  Sines to Solve Triangles
 Flash Card 8.2 Use Law of Cosines to Solve Triangles
 Flash Card 8.2 Use Law of Sines and Cosines to Solve Triangles
 If you have a text, see Section 8.2 - - 1-5, 7, 8, 32 - 35, 37, 41

Due Tuesday, Feb. 25, 11:59 pm:  E-HW 05 Section 7.6 -7.8
 Due Tuesday, Feb. 25, 11:59 pm:  E-HW 06 Section 8.1

 Writing Assignment 4: The Gateway Arch due Tuesday, Feb. 25

 Tuesday, Feb. 25: QUIZ 5 over Section 7.6-7.8
Class meeting Topic Assignment
Tuesday, Feb. 25 The Law of Cosines and the Ambiguous Case of the Law of Sines  Today's objectives:
 1. Solve for sides and angles of a triangle using the Law of Cosines.
 2. Determine when you can use the Law of Cosines and when you can use the Law of Sines. 
 3. Identify and solve problem situations involving the ambiguous case of the Law of Sines.
4. Convert coordinates from polar to rectangular and vice versa

Do (for practice):
Flash Card 8.2 Use Law of Cosines to Solve Triangles
 Flash Card 8.2 Use Law of Sines and Cosines to Solve Triangles
 Flash Card 8.2 Determine the number of triangles that are possible
 Flash Card 8.2 The Ambiguous Case
 Flash Card 8.3 Convert Polar => Rectangular (WITH or WITHOUT a calculator)
 Flash Card 8.3 Convert Rectangular=> Polar (WITH or WITHOUT a calculator)
 Flash Card 9.1 Solving Trig Equations Graphically
Optional: If you have a text, see Section 8.2  -- 6, 9-31,36,40, 42-44, 47, 48 and Section 8.3  -- 1-22

Due tonight, Tuesday, Feb. 25, 11:59 pm:  E-HW 05 Section 7.6 -7.8
 Due tonight, Tuesday, Feb. 25, 11:59 pm:  E-HW 06 Section 8.1
 Due Monday, March 3, 11:59 pm: E-HW 07 Section 8.2
 Due Monday, March 3, 11:59 pm: E-HW 08 Section 8.3 and 9.1

 Writing Assignment 5: The Law of Sines and Cosines to the Rescue due Thursday, March 19
Thursday, Feb. 27 Polar Coordinates (8.3) and Solving Trig Equations Graphically (9.1)

Today's objectives:
1. Convert coordinates from polar to rectangular and vice versa
2. Solve a trig equation graphically.
3. Rewrite trigonometric expressions.
4. Build fractional fluency.

 Do (for practice):
Flash Card 8.3 Convert Polar => Rectangular (WITH or WITHOUT a calculator)
 Flash Card 8.3 Convert Rectangular=> Polar (WITH or WITHOUT a calculator)
 Flash Card 9.1 Solving Trig Equations Graphically
Optional: Section 8.3  -- 1-22 and Section 9.1 -- 1-30, 38, 39, 41
 
Due Tuesday, March 3, 11:59 pm: E-HW 07 Section 8.2
 Due Tuesday, March 3, 11:59 pm: E-HW 08 Section 8.3 and 9.1

Tuesday, March 3: QUIZ 6 over Sections 8.1-8.3, 9.1

 Writing Assignment 5: The Law of Sines and Cosines to the Rescue due Thursday, March 19 (extended to March 26)
Class meeting Topic Assignment
Tuesday, March 3 Trigonometric Identities (9.2) Today's objectives:
1. Rewrite trigonometric expressions.
2. Build fractional fluency.

Do (for practice):
Flash Card 9.2 Trigonometric Identities
If you have a text, see Section 9.2  -- 1-12, 14, 16-26, 31, 32 and Worksheet (see BB).  For more see Section 7.7 -- 8-15
Due tonight, Tuesday, March 3, 11:59 pm: E-HW 07 Section 8.2
 Due tonight, Tuesday, March 3, 11:59 pm: E-HW 08 Section 8.3 and 9.1
Take Test 2 over Section 7.6, 7.7, 7.8, 8.1-8.3, and 9.1 in the Math Test Center during their hours this week, Monday, March 2 to Fri., March 6.  See the folder on BB called Review for Exams for more details.

Writing Assignment 5: The Law of Sines and Cosines to the Rescue due Thursday, March 19 (extended to 11:59 PM, March 26)
Wed., Mar. 4 Walk-in testing available. You can take Test 2 in the Math Test Center (KT G18) Wed., Mar. 4 from  9:00 AM - 7:00 PM.
(It closes at 8:00 pm)
Thursday, Mar. 5 No class meeting
Walk-in testing available.		 No class meeting,
You can take Test 2 in the Math Test Center (KT G18) Thurs., Mar. 5,  9:00 AM-7:00 PM
(It closes at 8:00 pm.)
Friday, Mar. 6 Walk-in testing available. You can take Test 2 in the Math Test Center (KT G18) Fri., Mar. 6,  9:00 AM - 3:30 PM
(It closes at 4:30 pm.)
This is the last day to take the test.
Class meeting Topic Assignment
March 9-13 Spring Break No class meetings.
Have a great Spring Break!
Class meeting Topic Assignment
March 16-20 Spring Break Continued
No class meetings.
Take another week off.
We are about to go on safari into the exciting world of remote learning.

See my announcement about this on Blackboard.


Rhinos, we are going on safari!

See Blackboard from here forward for learning activities and due dates
Week 9: March 23-29
Week 10: March 30-April 5
Week 11: April 6-12
Week 12: April 13-19
Week 13: April 20-26
Week 14: April 27 - May 3
Week 15: May 4-8  Final Exam Week

This page is on the Web at http://users.pfw.edu/lamaster/ma154/s20ma154.htm