An NSF Supported Initiative

 Department of Mathematical Sciences
Physics-Based Calculus
 A Workshop for Science and Mathematics Teachers
Friday, May 4, to Sunday, May 6, 2001.


IPFW Faculty Organizers
Adam Coffman
Timothy Grove
Peter Hamburger
Mark Masters
Yifei Pan

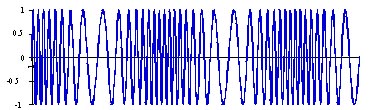 FM Radio signal
An FM Radio Signal

Mathematics Throughout The Curriculum Links
The MTC Page
IPFW Honors Calculus

We invite teachers of Mathematics or Science, at the high school or college level, to participate. The theme of the workshop will be Fourier Analysis, a branch of mathematics which has many applications, and which can be incorporated into a physics or calculus curriculum.

The 2001 Workshop has concluded! Participants should expect some materials and reimbursement to arrive in the mail.

PHOTOS FROM THE WORKSHOP

Schedule

  • The program is in Kettler Hall, on the IPFW campus.
  • Friday, May 4: start at 2:00, break at 4:00, stop at 6:00.
  • Demonstration of physics experiments: masses on vertical and horizontal springs.
  • Demonstration of software for physics, analyzing data from force and position detectors.
  • Discussion of Fourier coefficients, demonstration of Maple software.

  • Saturday, May 5: start at 9:00, lunch at 12:00, break at 3:00, stop at 5:00.
  • Demonstration of physics experiments: equipment for analyzing sound waves and vibrations, resonance frequencies of masses on springs, laser optics, the Michelson apparatus, and the Doppler effect.
  • Presentation on honors research in geology and mathematics, by IPFW student Alex James.
  • Hands-on physics activities, using the computer to analyze motion and sound data.
  • Demonstration of internet software for mathematics --- available on Professor Pan's Java Technology Laboratory web site.
  • Discussion of applications of Fourier analysis in biology, and the structure of Professor Hamburger's Honors Calculus course.

  • Sunday, May 6: start at 9:00, break at 11:00, stop at 12:00.
  • Discussion of complex numbers and the mathematical derivation of the Fourier transform.
  • Discussion of issues in the calculus curriculum.

One of the aims of the Mathematics Throughout the Curriculum project is the "dissemination" of its ideas. So, the project will provide funding for some (up to 30) workshop participants, in the amount of $150.

Registration

Registration is closed, but if you have questions about this workshop, please contact Professor Hamburger (Hamburge (at) ipfw.edu) or one of the organizers.

Some Applications of Fourier Analysis in Physics

Here are some brief descriptions of physics projects where both the theoretical predictions and the experimental data can be analyzed using Fourier methods.

  • Force and Motion; Vibrations and Spring Systems.

    Monitoring the force exerted on a probe at the upper support we can determine the modes of vibration in coupled oscillators.

    A graph of experimental data and its Fourier Transform (79KB)

    •  

  • Building vibration analysis.

    Using speakers and a simple vibration probe we can monitor and determine the modes and frequency of vibration.

    A photo (104KB) of the monitoring device

    •  

  • Sound analysis.

    The human voice and musical instruments can be examined using Fourier transforms to determine the frequency components and their relative phases.

    Waveform of a Reed.

    Fourier Transform of above waveform

    •  

  • Light : Double slit interference, Interferometers

    Splitting light such that the two parts travel different path lengths produces interference. The interference pattern can be considered as a Fourier transform on the incident light – dependent upon the wavelength of the light and the path difference between the two paths.

    A photo (356KB) of the laser experiment
    A graph with a curve and data points

    •  

  • Light : Fourier Optics

    Lenses, when arranged appropriately, take a two-dimensional Fourier transform of an object. This process can be used for image processing and filtering. This same process can be employed to produce calculated holograms.

     

Department of Mathematical Sciences
Indiana University - Purdue University Fort Wayne
phone: (219) 481-6821
BackTo the Department of Mathematical Sciences